

Next: Inclusion of Multiple Scattering
Up: Track Fitting in r-
Previous: Track Fitting in r-
We will show below that in the high momentum limit the effects of
multiple scattering can be implemented in a continuous manner.
For this purpose, it turns out very useful to set up
machinary to move the pivot, taking into account the effects of
multiple scattering.
We first consider the pivot transformation without multiple scattering.
The track parameter vector (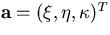 )transforms under an infinitesimal change of pivot:
)transforms under an infinitesimal change of pivot:
as follows:
This can be cast into a vector equation:
| 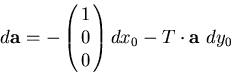 |
(42) |
where
| 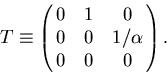 |
(43) |
From now on, we keep dx0 = 0![[*]](foot_motif.gif) , which reduces
the pivot transformation to a single parameter group and
the above equation to
, which reduces
the pivot transformation to a single parameter group and
the above equation to
| 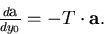 |
(44) |
Under this condition, 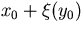 represents the x coordinate
of the track at y = y0.
In terms of the generator T, the transformation
matrix for the error matrix is given by
represents the x coordinate
of the track at y = y0.
In terms of the generator T, the transformation
matrix for the error matrix is given by
| 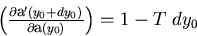 |
(45) |
which implies
| 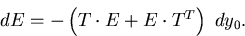 |
(46) |


Next: Inclusion of Multiple Scattering
Up: Track Fitting in r-
Previous: Track Fitting in r-
Keisuke Fujii
12/4/1998
![]() )transforms under an infinitesimal change of pivot:
)transforms under an infinitesimal change of pivot:
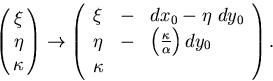
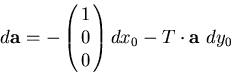
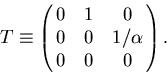
![[*]](foot_motif.gif) , which reduces
the pivot transformation to a single parameter group and
the above equation to
, which reduces
the pivot transformation to a single parameter group and
the above equation to