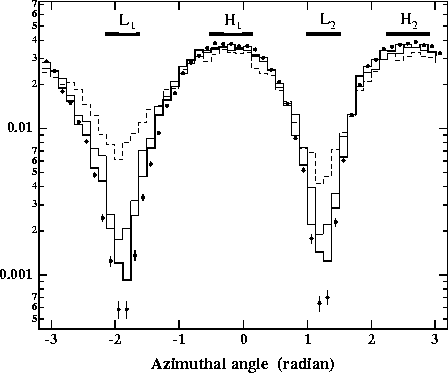
Figure 7:
Azimuthal angular distributions measured for 6< r < 7cm
at z=+1m for R=85.5(solid circles), 42.8(solid line), 28.5(dotted
one) and 8.55(dashed one) corresponding to ![]() ,
2
,
2![]() , 3
, 3![]() and 10
and 10![]() ,
respectively. The distributions are normalized by the total number of
particles. The angular regions of
,
respectively. The distributions are normalized by the total number of
particles. The angular regions of ![]() (left),
(left), ![]() (right),
(right),
![]() (down) and
(down) and ![]() (up) are also shown.
(up) are also shown.
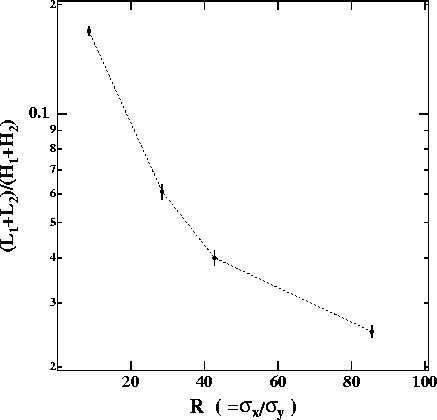
Figure 8:
Number of particles in ![]() and
and ![]() normalized by that
in
normalized by that
in ![]() and
and ![]() as a function of R. The same data are used as
in the previous figure. The errors are statistical, corresponding to
160 bunch crossings, except for the lowest R, which corresponds to
800 bunch crossings.
as a function of R. The same data are used as
in the previous figure. The errors are statistical, corresponding to
160 bunch crossings, except for the lowest R, which corresponds to
800 bunch crossings.
Figure 7 shows the azimuthal angular distributions
measured for 6< r < 7cm at z=+1m for various values of
![]() 's, where 4 angular regions are defined for further
numerical studies, i.e.
's, where 4 angular regions are defined for further
numerical studies, i.e. ![]() (left)
(left)![]() ,
,
![]() (right)
(right)![]() ,
, ![]() (down)
(down)![]() and
and ![]() (up)
(up)![]() . By comparing this
figure with Fig.4 we can clearly see that the
linear dependence on R of the yields in the depletion region is
preserved through the motion in the solenoid. The effect of the
horizontal crossing can still be seen in Fig.7; that is,
there is more depletion on the left side(
. By comparing this
figure with Fig.4 we can clearly see that the
linear dependence on R of the yields in the depletion region is
preserved through the motion in the solenoid. The effect of the
horizontal crossing can still be seen in Fig.7; that is,
there is more depletion on the left side(![]() ) than on the right
(
) than on the right
(![]() ). The numbers of particles are expected to be 2.4, 3.5, 120,
and 120 per single-bunch crossing in
). The numbers of particles are expected to be 2.4, 3.5, 120,
and 120 per single-bunch crossing in ![]() ,
, ![]() ,
, ![]() and
and ![]() ,
respectively, under the nominal beam condition. The statistics
will actually be much better because the beam pulse contains 72
bunches. Thus, there will be no problem in the statistical
sensitivity for this measurement, although the yields decrease
inversely proportional to
,
respectively, under the nominal beam condition. The statistics
will actually be much better because the beam pulse contains 72
bunches. Thus, there will be no problem in the statistical
sensitivity for this measurement, although the yields decrease
inversely proportional to ![]() . The ratio of
. The ratio of ![]() is plotted as a function of R in Fig.8.
Actually, this ratio depends only on R, as expected by
Eq.13. Since
is plotted as a function of R in Fig.8.
Actually, this ratio depends only on R, as expected by
Eq.13. Since ![]() is measured from
is measured from ![]() ,
we can obtain
,
we can obtain ![]() by this azimuthal angular
distribution. The statistical accuracy will be less than
0.1
by this azimuthal angular
distribution. The statistical accuracy will be less than
0.1![]() with a few pulse-train crossings if no
significant background exists.
with a few pulse-train crossings if no
significant background exists.