As stated earlier, a large number of low-energy electron-positron pairs are produced through beam-beam collisions. Out of these low-energy particles, we hereafter call those that have the same charge as the oncoming beam ``same-charge'' particles. Particles with the opposite charge are called ``opposite-charge'' particles.
Figure 14.6 has shown that ``same-charge'' particles generally experience large deflections during the collision process because of the strong electromagnetic force due to the oncoming beam. On the other hand, the ``opposite-charge'' particles tend to oscillate inside the beam size space of the oncoming beam, because of the focusing force that is exerted on them. Consequently, the ``opposite-charge'' particles are scattered at much smaller angles.
This phenomenon is well described by a scattering process of ![]() in a two-dimensional
Coulomb potential which is Lorentz-boosted to the rest frame of the oncoming
beam[10]. Since this potential is produced by the intense electric charge of the
oncoming beam, it is a function of the transverse size (
in a two-dimensional
Coulomb potential which is Lorentz-boosted to the rest frame of the oncoming
beam[10]. Since this potential is produced by the intense electric charge of the
oncoming beam, it is a function of the transverse size (![]() ,
,![]() ) and
intensity of that beam. Therefore, the distribution of deflected particles provides
information on the oncoming beam, particularly its angular distribution.
Hence, a measurement of the angular distribution of pair particles offers an excellent
opportunity for determining the beam profile at the IP.
) and
intensity of that beam. Therefore, the distribution of deflected particles provides
information on the oncoming beam, particularly its angular distribution.
Hence, a measurement of the angular distribution of pair particles offers an excellent
opportunity for determining the beam profile at the IP.
It should be noted that this technique allows one to measure the sizes of the two beams independently. This is because the low-energy particles are deflected asymmetrically in the forward and backward angular regions if the two beams have different beam parameters. It should be noted that there are two independent Coulomb potentials due to the two beams that are separated by a large relative Lorentz-boost along the beam axis. With a sufficiently accurate measurement of low-energy particle distributions, we can also measure the relative displacement and transverse rotation of two beams. In addition, this measurement can provide signals that can be used for a real-time, fast feedback to maintain the collision operation of the linear collider without disrupting the data collection by the experimental facility.
The basic characteristics and expected performance of this beam profile measurement technique has been extensively discussed in a published paper[11]. Here, we show some results from simulations with the JIM code.
We use the monitors that are located at ![]() m (both sides) from the IP. They are
arranged as shown in Figure 14.7. Each monitor consists of two layers of disk-shaped
detectors. Each disk constitutes a pixel device which is made of 300
m (both sides) from the IP. They are
arranged as shown in Figure 14.7. Each monitor consists of two layers of disk-shaped
detectors. Each disk constitutes a pixel device which is made of 300![]() m-thick silicon.
The monitor can measure both the radial position (3 < r < 12 cm) and the azimuthal angles
(
m-thick silicon.
The monitor can measure both the radial position (3 < r < 12 cm) and the azimuthal angles
(![]() ) of the traversing particles with pixels of
) of the traversing particles with pixels of ![]() m
m![]() channel size. In addition, the pixel detectors will be arranged so that they can also
measure the energy deposit left by the particles.
channel size. In addition, the pixel detectors will be arranged so that they can also
measure the energy deposit left by the particles.
Figure 14.13 shows the simulated distributions of hits as measured by the two disks of the monitor at z=1 m. Without taking specific measures to reduce the background, asymmetric angular distributions can be clearly seen over ``uniform" backgrounds. The ``maximum" radial distance can be found at around r=5.5 cm.
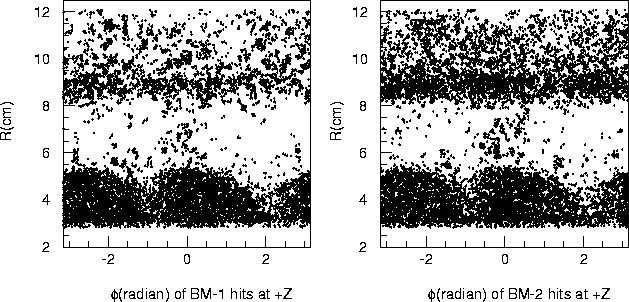
Figure 14.13: Hit distributions observed at two disks of the beam profile monitor at z=1m for 10
bunch-crossings. BM-2 is behind BM-1, as viewed from the IP.
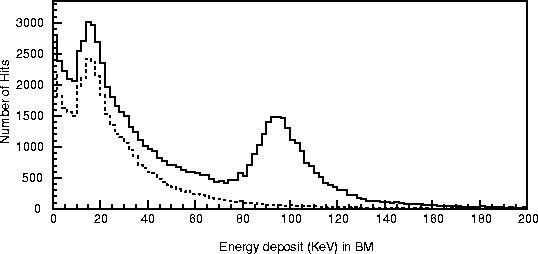
Figure 14.14: Energy deposits observed at the beam profile monitor for 10 bunch-crossings. The
solid and dotted lines are for the total and secondary particles, respectively.
Figure 14.14 shows the distribution of energy deposit on the beam profile monitor. The well-separated peak in this figure around 90 keV comprises the primary particles traversing the monitor, while peak around a lower energy deposit of 20 keV is created by the secondary particles which are backscattered from the QC1 and the luminosity monitor. It is seen that the energy deposits are very effective to discriminate the backgrounds. By selecting the hits associated with energy deposits > 70 keV, the signals of interest are significantly enhanced, as shown in Figure 14.15.
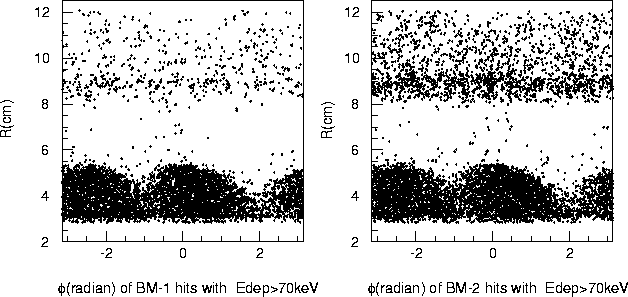
Figure 14.15: Distribution of hits whose energy deposit exceeds 70keV, as observed at the
two disks of the beam profile monitor at z=1 m for 10 bunch-crossings.
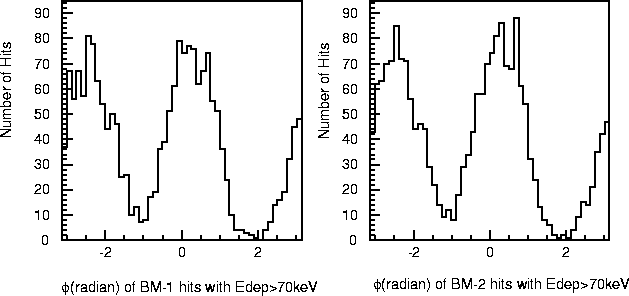
Figure 14.16: Angular distributions in 4.5 < r < 5.5cm for energy deposits of more than
70keV, observed at two disks of the beam profile monitor at z=1m for 10 bunch-crossings.
Figure 14.16 shows the hit angular distributions in the region 4.5 < r <5.5 cm. The hit pattern in this region carries information that is sensitive to the aspect ratio of the transverse beam sizes. As can be clearly seen in these figures, the asymmetric angular distribution can be measured very well with a very small amount of background.
These results indicate that the beam profile monitor based on measurements of low energy pair particles is a very promising technique.