




Next: Usage
Up: Breit-Wheeler Process
Previous: Breit-Wheeler Process
- k,
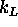 ,p,p'
,p,p'
- 4-momenta of the initial photon, laser photon,
final electron and positron.
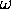 ,
,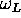 ,
, ,
,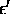
-
Energies of the initial photon, laser photon,
final electron and positron.
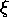
- Laser intensity parameter
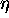
- Laser energy parameter:
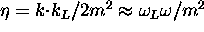 .
.
- n
- Number of absorbed laser photons
- x
- =
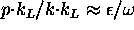 . (0<x<1)
. (0<x<1)
- u
- u=1/[4x(1-x)],
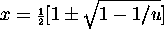 , (
, (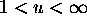 ).
).

- Maximum u for given n:
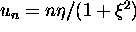 .
.
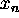
-

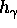
- Initial photon helicity

- Laser helicity
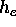
- Final electron helicity
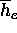
- `Detector' helicity of the final electron.
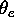
- Final electron angle
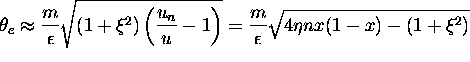
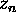
- The argument of the Bessel functions in the following
expressions:
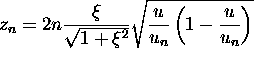
Total number of pair electrons per unit time summed over the positron
polarization is
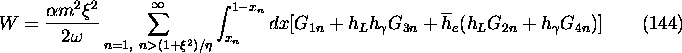
Sum over final electron polarization gives
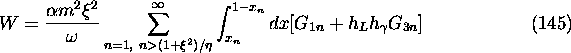
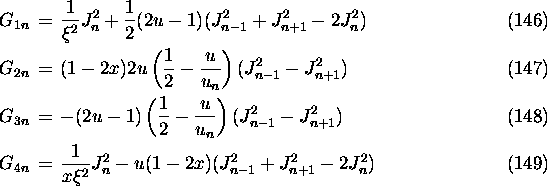
These formulas can be obtained from those of  by the replacement
by the replacement
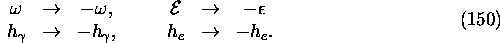
This implies
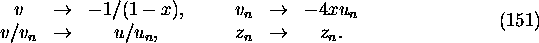
For convenience, we have changed the sign as
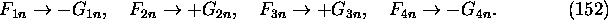
For given x and n, the final momenta are given by
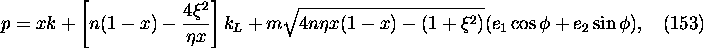

Here, 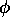 is the azimuthal scattering angle in a head-on frame and
is the azimuthal scattering angle in a head-on frame and
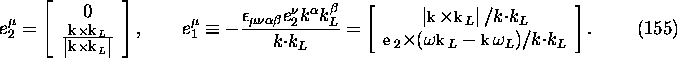
These vectors satisfy

Toshiaki Tauchi
Thu Dec 3 17:27:26 JST 1998
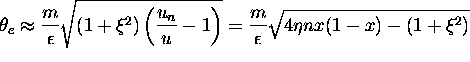
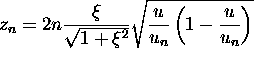
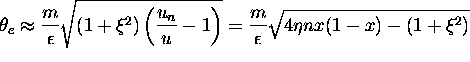
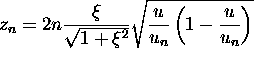
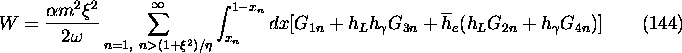
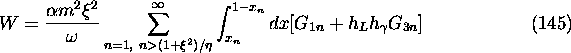
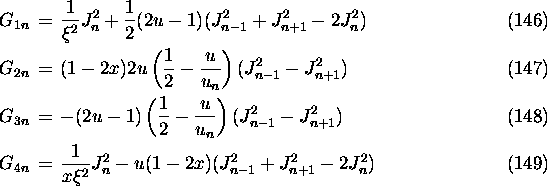
![]() by the replacement
by the replacement
![]()
![]()
![]()
![]()
![]()
![]() is the azimuthal scattering angle in a head-on frame and
is the azimuthal scattering angle in a head-on frame and
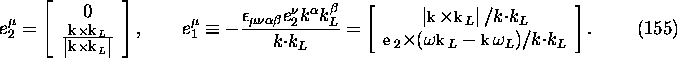
![]()